「《交渉ゲーム》」の版間の差分
H.Yamaguchi (トーク | 投稿記録) |
|||
| (3人の利用者による、間の4版が非表示) | |||
| 3行目: | 3行目: | ||
交渉は, 複数の当事者が協力の条件を協議する状況であり, 各自が相互依存関係の中で意思決定をするゲームの状況である. [[交渉ゲーム]] (bargaining game) の研究は, ナッシュ (J. F. Nash)による[[2人交渉問題]]の交渉解に始まり, 近年では, ルビンシュタイン (A. Rubinstein) の[[交互オファーゲーム]]による交渉過程の分析が代表的である. | 交渉は, 複数の当事者が協力の条件を協議する状況であり, 各自が相互依存関係の中で意思決定をするゲームの状況である. [[交渉ゲーム]] (bargaining game) の研究は, ナッシュ (J. F. Nash)による[[2人交渉問題]]の交渉解に始まり, 近年では, ルビンシュタイン (A. Rubinstein) の[[交互オファーゲーム]]による交渉過程の分析が代表的である. | ||
| − | ''' | + | '''1 2人交渉問題と交渉解''' 交渉の結果は, 妥結して協力するか決裂するかであり, 交渉の妥結点は交渉決裂時の状態に依存する. 2人交渉問題は, 2人のプレイヤー間の交渉を, 協力実現可能集合 <math>S\, </math> と交渉の基準点 <math>d\in S\, </math> の組 <math>(S, d)\, </math> として記述する. <math>S\, </math>は2次元実数ベクトル空間の部分集合である. 以下では, ベクトル間の不等号は要素ごとの不等号を意味する. |
| − | 協力実現可能集合 <math>S\, </math> は2人が協力して実現可能な[[利得 (ゲームの)|利得]]ベクトル集合であり, 妥結点の候補を表す. 厳密には, 2人のプレイヤーの[[相関戦略]]により実現可能な[[フォンノイマン・モルゲンシュテルン期待効用]]ベクトル <math>(u_{1}, u_{2})\, </math> の集合が <math>S\, </math> である. 交渉問題では, 交渉決裂時は, 各プレイヤーは予め想定された行動を独立に実行し, 交渉の基準点 <math>d=(d_{1}, d_{2})\, </math> の利得を得るとする. 集合 <math>I(S, d)=\{u \in S|u \ge d\}\, </math> を (個人合理的) 交渉領域と呼ぶ. 通常, (1) 集合 <math>S\, </math> がコンパクト凸であり, (2) <math>x>d\, </math> なる点 <math>x \in S\, </math> が存在する, という2条件を満たす交渉問題が考察対象とされ, その集合を <math>B_{0}\, </math> とする. また, (1), (2)に加えて, 「<math>x \in S\, </math> かつ <math>x\ge y\ge d\, </math> ならば, <math>y\in S\, </math>」であり, 「交渉領域の[[弱パレート最適]]な境界線が水平, 垂直部分を持たない」交渉問題の集合を <math>B_{E}\, </math> とする. | + | 協力実現可能集合 <math>S\, </math> は2人が協力して実現可能な[[利得 (ゲームの)|利得]]ベクトル集合であり, 妥結点の候補を表す. 厳密には, 2人のプレイヤーの[[相関戦略]]により実現可能な[[フォンノイマン・モルゲンシュテルン効用関数|フォンノイマン・モルゲンシュテルン期待効用]]ベクトル <math>(u_{1}, u_{2})\, </math> の集合が <math>S\, </math> である. 交渉問題では, 交渉決裂時は, 各プレイヤーは予め想定された行動を独立に実行し, 交渉の基準点 <math>d=(d_{1}, d_{2})\, </math> の利得を得るとする. 集合 <math>I(S, d)=\{u \in S|u \ge d\}\, </math> を (個人合理的) 交渉領域と呼ぶ. 通常, (1) 集合 <math>S\, </math> がコンパクト凸であり, (2) <math>x>d\, </math> なる点 <math>x \in S\, </math> が存在する, という2条件を満たす交渉問題が考察対象とされ, その集合を <math>B_{0}\, </math> とする. また, (1), (2)に加えて, 「<math>x \in S\, </math> かつ <math>x\ge y\ge d\, </math> ならば, <math>y\in S\, </math>」であり, 「交渉領域の[[パレート最適|弱パレート最適]]な境界線が水平, 垂直部分を持たない」交渉問題の集合を <math>B_{E}\, </math> とする. |
交渉問題の集合 <math>B\, </math> に属す任意の交渉問題 <math>(S, d)\, </math> に, 妥結点 <math>a\in S\, </math> を与える関数 <math>f:B \to {\mathbf{ R}}^{2}\, </math> を, (<math>B\, </math> 上の)[[交渉解]] (bargaining solution) <math>f\, </math> という. 交渉解は妥結方法を示す概念である. ナッシュは合理的妥結方法が満たすべき4つの公理を挙げて, それらを満たす交渉解を分析した. | 交渉問題の集合 <math>B\, </math> に属す任意の交渉問題 <math>(S, d)\, </math> に, 妥結点 <math>a\in S\, </math> を与える関数 <math>f:B \to {\mathbf{ R}}^{2}\, </math> を, (<math>B\, </math> 上の)[[交渉解]] (bargaining solution) <math>f\, </math> という. 交渉解は妥結方法を示す概念である. ナッシュは合理的妥結方法が満たすべき4つの公理を挙げて, それらを満たす交渉解を分析した. | ||
| 21行目: | 21行目: | ||
| − | <center><table><tr><td align=center>[[画像:0075-a-g-07f1-mof. | + | <center><table><tr><td align=center>[[画像:0075-a-g-07f1-mof.png|center|図1:交渉問題の妥結点]]</td></tr> |
<td align=center>図1:交渉問題の妥結点</td></table></center> | <td align=center>図1:交渉問題の妥結点</td></table></center> | ||
| 91行目: | 91行目: | ||
| − | <center><table><tr><td align=center>[[画像:0075-a-g-07f2-mof. | + | <center><table><tr><td align=center>[[画像:0075-a-g-07f2-mof.png|center|図2:財分配の実現可能集合]]</td></tr> |
<td align=center>図2:財分配の実現可能集合</td></table></center> | <td align=center>図2:財分配の実現可能集合</td></table></center> | ||
| 117行目: | 117行目: | ||
[3] J. Nash, "The Bargaining Problem," ''Econometrica'', '''18''' (1950), 155-162. | [3] J. Nash, "The Bargaining Problem," ''Econometrica'', '''18''' (1950), 155-162. | ||
| + | |||
| + | [[category:ゲーム理論|こうしょうげーむ]] | ||
2007年8月9日 (木) 13:07時点における最新版
【こうしょうげーむ (bargaining game) 】
交渉は, 複数の当事者が協力の条件を協議する状況であり, 各自が相互依存関係の中で意思決定をするゲームの状況である. 交渉ゲーム (bargaining game) の研究は, ナッシュ (J. F. Nash)による2人交渉問題の交渉解に始まり, 近年では, ルビンシュタイン (A. Rubinstein) の交互オファーゲームによる交渉過程の分析が代表的である.
1 2人交渉問題と交渉解 交渉の結果は, 妥結して協力するか決裂するかであり, 交渉の妥結点は交渉決裂時の状態に依存する. 2人交渉問題は, 2人のプレイヤー間の交渉を, 協力実現可能集合 構文解析に失敗 (MathML、ただし動作しない場合はSVGかPNGで代替(最新ブラウザーや補助ツールに推奨): サーバー「https://en.wikipedia.org/api/rest_v1/」から無効な応答 ("Math extension cannot connect to Restbase."):): {\displaystyle S\, } と交渉の基準点 構文解析に失敗 (MathML、ただし動作しない場合はSVGかPNGで代替(最新ブラウザーや補助ツールに推奨): サーバー「https://en.wikipedia.org/api/rest_v1/」から無効な応答 ("Math extension cannot connect to Restbase."):): {\displaystyle d\in S\, } の組 として記述する. は2次元実数ベクトル空間の部分集合である. 以下では, ベクトル間の不等号は要素ごとの不等号を意味する.
協力実現可能集合 構文解析に失敗 (MathML、ただし動作しない場合はSVGかPNGで代替(最新ブラウザーや補助ツールに推奨): サーバー「https://en.wikipedia.org/api/rest_v1/」から無効な応答 ("Math extension cannot connect to Restbase."):): {\displaystyle S\, } は2人が協力して実現可能な利得ベクトル集合であり, 妥結点の候補を表す. 厳密には, 2人のプレイヤーの相関戦略により実現可能なフォンノイマン・モルゲンシュテルン期待効用ベクトル 構文解析に失敗 (MathML、ただし動作しない場合はSVGかPNGで代替(最新ブラウザーや補助ツールに推奨): サーバー「https://en.wikipedia.org/api/rest_v1/」から無効な応答 ("Math extension cannot connect to Restbase."):): {\displaystyle (u_{1}, u_{2})\, } の集合が 構文解析に失敗 (MathML、ただし動作しない場合はSVGかPNGで代替(最新ブラウザーや補助ツールに推奨): サーバー「https://en.wikipedia.org/api/rest_v1/」から無効な応答 ("Math extension cannot connect to Restbase."):): {\displaystyle S\, } である. 交渉問題では, 交渉決裂時は, 各プレイヤーは予め想定された行動を独立に実行し, 交渉の基準点 構文解析に失敗 (MathML、ただし動作しない場合はSVGかPNGで代替(最新ブラウザーや補助ツールに推奨): サーバー「https://en.wikipedia.org/api/rest_v1/」から無効な応答 ("Math extension cannot connect to Restbase."):): {\displaystyle d=(d_{1}, d_{2})\, } の利得を得るとする. 集合 構文解析に失敗 (MathML、ただし動作しない場合はSVGかPNGで代替(最新ブラウザーや補助ツールに推奨): サーバー「https://en.wikipedia.org/api/rest_v1/」から無効な応答 ("Math extension cannot connect to Restbase."):): {\displaystyle I(S, d)=\{u \in S|u \ge d\}\, } を (個人合理的) 交渉領域と呼ぶ. 通常, (1) 集合 構文解析に失敗 (MathML、ただし動作しない場合はSVGかPNGで代替(最新ブラウザーや補助ツールに推奨): サーバー「https://en.wikipedia.org/api/rest_v1/」から無効な応答 ("Math extension cannot connect to Restbase."):): {\displaystyle S\, } がコンパクト凸であり, (2) 構文解析に失敗 (MathML、ただし動作しない場合はSVGかPNGで代替(最新ブラウザーや補助ツールに推奨): サーバー「https://en.wikipedia.org/api/rest_v1/」から無効な応答 ("Math extension cannot connect to Restbase."):): {\displaystyle x>d\, } なる点 構文解析に失敗 (MathML、ただし動作しない場合はSVGかPNGで代替(最新ブラウザーや補助ツールに推奨): サーバー「https://en.wikipedia.org/api/rest_v1/」から無効な応答 ("Math extension cannot connect to Restbase."):): {\displaystyle x \in S\, } が存在する, という2条件を満たす交渉問題が考察対象とされ, その集合を 構文解析に失敗 (MathML、ただし動作しない場合はSVGかPNGで代替(最新ブラウザーや補助ツールに推奨): サーバー「https://en.wikipedia.org/api/rest_v1/」から無効な応答 ("Math extension cannot connect to Restbase."):): {\displaystyle B_{0}\, } とする. また, (1), (2)に加えて, 「構文解析に失敗 (MathML、ただし動作しない場合はSVGかPNGで代替(最新ブラウザーや補助ツールに推奨): サーバー「https://en.wikipedia.org/api/rest_v1/」から無効な応答 ("Math extension cannot connect to Restbase."):): {\displaystyle x \in S\, } かつ ならば, 」であり, 「交渉領域の弱パレート最適な境界線が水平, 垂直部分を持たない」交渉問題の集合を とする.
交渉問題の集合 構文解析に失敗 (MathML、ただし動作しない場合はSVGかPNGで代替(最新ブラウザーや補助ツールに推奨): サーバー「https://en.wikipedia.org/api/rest_v1/」から無効な応答 ("Math extension cannot connect to Restbase."):): {\displaystyle B\, } に属す任意の交渉問題 構文解析に失敗 (MathML、ただし動作しない場合はSVGかPNGで代替(最新ブラウザーや補助ツールに推奨): サーバー「https://en.wikipedia.org/api/rest_v1/」から無効な応答 ("Math extension cannot connect to Restbase."):): {\displaystyle (S, d)\, } に, 妥結点 構文解析に失敗 (MathML、ただし動作しない場合はSVGかPNGで代替(最新ブラウザーや補助ツールに推奨): サーバー「https://en.wikipedia.org/api/rest_v1/」から無効な応答 ("Math extension cannot connect to Restbase."):): {\displaystyle a\in S\, } を与える関数 構文解析に失敗 (MathML、ただし動作しない場合はSVGかPNGで代替(最新ブラウザーや補助ツールに推奨): サーバー「https://en.wikipedia.org/api/rest_v1/」から無効な応答 ("Math extension cannot connect to Restbase."):): {\displaystyle f:B \to {\mathbf{ R}}^{2}\, } を, (構文解析に失敗 (MathML、ただし動作しない場合はSVGかPNGで代替(最新ブラウザーや補助ツールに推奨): サーバー「https://en.wikipedia.org/api/rest_v1/」から無効な応答 ("Math extension cannot connect to Restbase."):): {\displaystyle B\, } 上の)交渉解 (bargaining solution) 構文解析に失敗 (MathML、ただし動作しない場合はSVGかPNGで代替(最新ブラウザーや補助ツールに推奨): サーバー「https://en.wikipedia.org/api/rest_v1/」から無効な応答 ("Math extension cannot connect to Restbase."):): {\displaystyle f\, } という. 交渉解は妥結方法を示す概念である. ナッシュは合理的妥結方法が満たすべき4つの公理を挙げて, それらを満たす交渉解を分析した.
公理1 (正アフィン変換からの独立性). 交渉問題 構文解析に失敗 (MathML、ただし動作しない場合はSVGかPNGで代替(最新ブラウザーや補助ツールに推奨): サーバー「https://en.wikipedia.org/api/rest_v1/」から無効な応答 ("Math extension cannot connect to Restbase."):): {\displaystyle (S, d)\, } と 構文解析に失敗 (MathML、ただし動作しない場合はSVGかPNGで代替(最新ブラウザーや補助ツールに推奨): サーバー「https://en.wikipedia.org/api/rest_v1/」から無効な応答 ("Math extension cannot connect to Restbase."):): {\displaystyle (S^{\prime}, d^{\prime})\, } が, ある正アフィン変換 構文解析に失敗 (MathML、ただし動作しない場合はSVGかPNGで代替(最新ブラウザーや補助ツールに推奨): サーバー「https://en.wikipedia.org/api/rest_v1/」から無効な応答 ("Math extension cannot connect to Restbase."):): {\displaystyle y=(c_{1}x_{1}+b_{1}, c_{2}x_{2}+b_{2}), c_{1}, c_{2}>0\, } , により一致するとき, 交渉解が両問題に与える妥結点もその変換の下で一致する.
公理2 (パレート最適性). 交渉解はパレート最適な妥結点を与える.
公理3 (対称性). 交渉問題 構文解析に失敗 (MathML、ただし動作しない場合はSVGかPNGで代替(最新ブラウザーや補助ツールに推奨): サーバー「https://en.wikipedia.org/api/rest_v1/」から無効な応答 ("Math extension cannot connect to Restbase."):): {\displaystyle (S, d)\, } が対称的で, 構文解析に失敗 (MathML、ただし動作しない場合はSVGかPNGで代替(最新ブラウザーや補助ツールに推奨): サーバー「https://en.wikipedia.org/api/rest_v1/」から無効な応答 ("Math extension cannot connect to Restbase."):): {\displaystyle (x, y)\in S\Leftrightarrow(y, x)\in S\, } , かつ, 構文解析に失敗 (MathML、ただし動作しない場合はSVGかPNGで代替(最新ブラウザーや補助ツールに推奨): サーバー「https://en.wikipedia.org/api/rest_v1/」から無効な応答 ("Math extension cannot connect to Restbase."):): {\displaystyle d_{1}=d_{2}\, } ならば, 交渉解の与える妥結点 構文解析に失敗 (MathML、ただし動作しない場合はSVGかPNGで代替(最新ブラウザーや補助ツールに推奨): サーバー「https://en.wikipedia.org/api/rest_v1/」から無効な応答 ("Math extension cannot connect to Restbase."):): {\displaystyle (a_{1}, a_{2})\, } も対称的で, 構文解析に失敗 (MathML、ただし動作しない場合はSVGかPNGで代替(最新ブラウザーや補助ツールに推奨): サーバー「https://en.wikipedia.org/api/rest_v1/」から無効な応答 ("Math extension cannot connect to Restbase."):): {\displaystyle a_{1}=a_{2}\, } .
公理4 (無関連な代替案からの独立性). 基準点が等しい交渉問題 構文解析に失敗 (MathML、ただし動作しない場合はSVGかPNGで代替(最新ブラウザーや補助ツールに推奨): サーバー「https://en.wikipedia.org/api/rest_v1/」から無効な応答 ("Math extension cannot connect to Restbase."):): {\displaystyle (S, d)\, } と 構文解析に失敗 (MathML、ただし動作しない場合はSVGかPNGで代替(最新ブラウザーや補助ツールに推奨): サーバー「https://en.wikipedia.org/api/rest_v1/」から無効な応答 ("Math extension cannot connect to Restbase."):): {\displaystyle (T, d)\, } について, 構文解析に失敗 (MathML、ただし動作しない場合はSVGかPNGで代替(最新ブラウザーや補助ツールに推奨): サーバー「https://en.wikipedia.org/api/rest_v1/」から無効な応答 ("Math extension cannot connect to Restbase."):): {\displaystyle T\subseteq S\, } かつ 構文解析に失敗 (MathML、ただし動作しない場合はSVGかPNGで代替(最新ブラウザーや補助ツールに推奨): サーバー「https://en.wikipedia.org/api/rest_v1/」から無効な応答 ("Math extension cannot connect to Restbase."):): {\displaystyle f(S, d)\in T\, } ならば, 構文解析に失敗 (MathML、ただし動作しない場合はSVGかPNGで代替(最新ブラウザーや補助ツールに推奨): サーバー「https://en.wikipedia.org/api/rest_v1/」から無効な応答 ("Math extension cannot connect to Restbase."):): {\displaystyle f(T, d)=f(S, d)\, } .
公理1は利得の高低やその変化分の大小をプレイヤー間で比較する「個人間効用比較」の排除を求める公理であり, フォンノイマン・モルゲンシュテルン効用が正アフィン変換の下で同値なことからも仮定される. 公理2は交渉結果の効率性を求め, 公理3は, 交渉状況が対称的ならば妥結点も対称的であることを求めている. 公理4は, 妥結点とならなかった代替案を除いて, 再び交渉し直しても妥結点は変わらないことを求める公理である. ナッシュは, 交渉問題の集合 構文解析に失敗 (MathML、ただし動作しない場合はSVGかPNGで代替(最新ブラウザーや補助ツールに推奨): サーバー「https://en.wikipedia.org/api/rest_v1/」から無効な応答 ("Math extension cannot connect to Restbase."):): {\displaystyle B_{0}\, }
上で, 公理1-4を満たす交渉解が一意に定まることを証明した. ナッシュ解と呼ばれるその交渉解 構文解析に失敗 (MathML、ただし動作しない場合はSVGかPNGで代替(最新ブラウザーや補助ツールに推奨): サーバー「https://en.wikipedia.org/api/rest_v1/」から無効な応答 ("Math extension cannot connect to Restbase."):): {\displaystyle f^{{\rm N}}\, }
は, 交渉領域内で2人のプレイヤーの基準点からの利得増加分の積を最大化する点を妥結点とし, 構文解析に失敗 (MathML、ただし動作しない場合はSVGかPNGで代替(最新ブラウザーや補助ツールに推奨): サーバー「https://en.wikipedia.org/api/rest_v1/」から無効な応答 ("Math extension cannot connect to Restbase."):): {\displaystyle f^{\rm N}(S, d)= {\rm argmax}_{u\in I(S, d)}(u_{1}-d_{1})(u_{2}-d_{2})\, }
で与えられる [3].
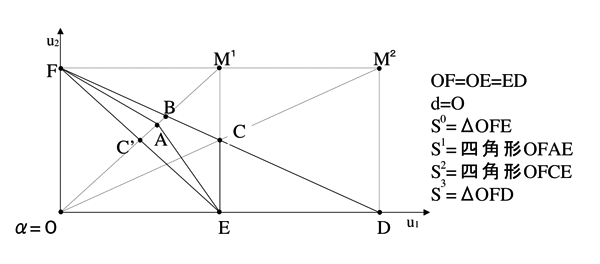
| 図1:交渉問題の妥結点 |
ナッシュ解の妥結点は, 公理系では仮定されないが, 個人合理的である. 図1は, ナッシュ解の妥結点と各公理の関係を示している. まず, 対称的交渉問題では, 公理2, 3から, 構文解析に失敗 (MathML、ただし動作しない場合はSVGかPNGで代替(最新ブラウザーや補助ツールに推奨): サーバー「https://en.wikipedia.org/api/rest_v1/」から無効な応答 ("Math extension cannot connect to Restbase."):): {\displaystyle f^{{\rm N}}(S^{0}, d)=C^{\prime}\, }
, 構文解析に失敗 (MathML、ただし動作しない場合はSVGかPNGで代替(最新ブラウザーや補助ツールに推奨): サーバー「https://en.wikipedia.org/api/rest_v1/」から無効な応答 ("Math extension cannot connect to Restbase."):): {\displaystyle f^{{\rm N}}(S^{1}, d)=A\, }
となる. 次に, 構文解析に失敗 (MathML、ただし動作しない場合はSVGかPNGで代替(最新ブラウザーや補助ツールに推奨): サーバー「https://en.wikipedia.org/api/rest_v1/」から無効な応答 ("Math extension cannot connect to Restbase."):): {\displaystyle f^{{\rm N}}(S^{0}, d)=C^{\prime}\, }
ならば, 公理1から, 構文解析に失敗 (MathML、ただし動作しない場合はSVGかPNGで代替(最新ブラウザーや補助ツールに推奨): サーバー「https://en.wikipedia.org/api/rest_v1/」から無効な応答 ("Math extension cannot connect to Restbase."):): {\displaystyle f^{{\rm N}}(S^{3}, d)=C\, }
となる. そして公理4から, 構文解析に失敗 (MathML、ただし動作しない場合はSVGかPNGで代替(最新ブラウザーや補助ツールに推奨): サーバー「https://en.wikipedia.org/api/rest_v1/」から無効な応答 ("Math extension cannot connect to Restbase."):): {\displaystyle f^{{\rm N}}(S^{2}, d)=f^{{\rm N}}(S^{3}, d)=C\, }
となる. 問題 構文解析に失敗 (MathML、ただし動作しない場合はSVGかPNGで代替(最新ブラウザーや補助ツールに推奨): サーバー「https://en.wikipedia.org/api/rest_v1/」から無効な応答 ("Math extension cannot connect to Restbase."):): {\displaystyle (S^{2}, d)\, }
は問題 構文解析に失敗 (MathML、ただし動作しない場合はSVGかPNGで代替(最新ブラウザーや補助ツールに推奨): サーバー「https://en.wikipedia.org/api/rest_v1/」から無効な応答 ("Math extension cannot connect to Restbase."):): {\displaystyle (S^{1}, d)\, }
よりも交渉領域が広いが, プレイヤー2の妥結点利得は減少している. よって, ナッシュ解の妥結点は単調的には推移しない.
個人間効用比較を排除する公理1の下で, 妥結点の単調性を求めた交渉解として, カライ・スモルディンスキー解 (Kalai-Smorodinsky solution, 以下構文解析に失敗 (MathML、ただし動作しない場合はSVGかPNGで代替(最新ブラウザーや補助ツールに推奨): サーバー「https://en.wikipedia.org/api/rest_v1/」から無効な応答 ("Math extension cannot connect to Restbase."):): {\displaystyle {\rm KS}\, } 解と略す) がある. 構文解析に失敗 (MathML、ただし動作しない場合はSVGかPNGで代替(最新ブラウザーや補助ツールに推奨): サーバー「https://en.wikipedia.org/api/rest_v1/」から無効な応答 ("Math extension cannot connect to Restbase."):): {\displaystyle {\rm KS}\, } 解では, 基準点に加え, 交渉の理想点 (各プレイヤー 構文解析に失敗 (MathML、ただし動作しない場合はSVGかPNGで代替(最新ブラウザーや補助ツールに推奨): サーバー「https://en.wikipedia.org/api/rest_v1/」から無効な応答 ("Math extension cannot connect to Restbase."):): {\displaystyle i\, } が交渉領域で獲得できる最大利得 構文解析に失敗 (MathML、ただし動作しない場合はSVGかPNGで代替(最新ブラウザーや補助ツールに推奨): サーバー「https://en.wikipedia.org/api/rest_v1/」から無効な応答 ("Math extension cannot connect to Restbase."):): {\displaystyle m_{i}={\rm max}\{u_{i}|u\in I(S, d)\}\, } の組 構文解析に失敗 (MathML、ただし動作しない場合はSVGかPNGで代替(最新ブラウザーや補助ツールに推奨): サーバー「https://en.wikipedia.org/api/rest_v1/」から無効な応答 ("Math extension cannot connect to Restbase."):): {\displaystyle M=(m_{1}, m_{2})\, } ) が考慮される. いま, 「基準点と理想点が共に等しい問題 構文解析に失敗 (MathML、ただし動作しない場合はSVGかPNGで代替(最新ブラウザーや補助ツールに推奨): サーバー「https://en.wikipedia.org/api/rest_v1/」から無効な応答 ("Math extension cannot connect to Restbase."):): {\displaystyle (S, d)\, } と 構文解析に失敗 (MathML、ただし動作しない場合はSVGかPNGで代替(最新ブラウザーや補助ツールに推奨): サーバー「https://en.wikipedia.org/api/rest_v1/」から無効な応答 ("Math extension cannot connect to Restbase."):): {\displaystyle (T, d)\, } について, ならば, 構文解析に失敗 (MathML、ただし動作しない場合はSVGかPNGで代替(最新ブラウザーや補助ツールに推奨): サーバー「https://en.wikipedia.org/api/rest_v1/」から無効な応答 ("Math extension cannot connect to Restbase."):): {\displaystyle f(S, d) \ge f(T, d)\, } 」という条件を限定単調性の公理と呼ぶと, 交渉問題の集合 構文解析に失敗 (MathML、ただし動作しない場合はSVGかPNGで代替(最新ブラウザーや補助ツールに推奨): サーバー「https://en.wikipedia.org/api/rest_v1/」から無効な応答 ("Math extension cannot connect to Restbase."):): {\displaystyle B_{0}\, } 上で, 公理1-3, かつ, 限定単調性を満たす交渉解が一意に定まる [2]. この解が構文解析に失敗 (MathML、ただし動作しない場合はSVGかPNGで代替(最新ブラウザーや補助ツールに推奨): サーバー「https://en.wikipedia.org/api/rest_v1/」から無効な応答 ("Math extension cannot connect to Restbase."):): {\displaystyle {\rm KS}\, } 解であり, 基準点と理想点を結ぶ線分と交渉領域のパレート最適な境界線との交点を妥結点とする. 以下, 構文解析に失敗 (MathML、ただし動作しない場合はSVGかPNGで代替(最新ブラウザーや補助ツールに推奨): サーバー「https://en.wikipedia.org/api/rest_v1/」から無効な応答 ("Math extension cannot connect to Restbase."):): {\displaystyle {\rm KS}\, } 解を 構文解析に失敗 (MathML、ただし動作しない場合はSVGかPNGで代替(最新ブラウザーや補助ツールに推奨): サーバー「https://en.wikipedia.org/api/rest_v1/」から無効な応答 ("Math extension cannot connect to Restbase."):): {\displaystyle f^{{\rm KS}}\, } で表す.
ナッシュ解と 構文解析に失敗 (MathML、ただし動作しない場合はSVGかPNGで代替(最新ブラウザーや補助ツールに推奨): サーバー「https://en.wikipedia.org/api/rest_v1/」から無効な応答 ("Math extension cannot connect to Restbase."):): {\displaystyle {\rm KS}\, } 解の一意性から, 公理4と限定単調性の公理は両立しない. これは, ナッシュ解と構文解析に失敗 (MathML、ただし動作しない場合はSVGかPNGで代替(最新ブラウザーや補助ツールに推奨): サーバー「https://en.wikipedia.org/api/rest_v1/」から無効な応答 ("Math extension cannot connect to Restbase."):): {\displaystyle {\rm KS}\, } 解が, 異なる観点から各々合理的な妥結方法であることを示す. 先の問題 構文解析に失敗 (MathML、ただし動作しない場合はSVGかPNGで代替(最新ブラウザーや補助ツールに推奨): サーバー「https://en.wikipedia.org/api/rest_v1/」から無効な応答 ("Math extension cannot connect to Restbase."):): {\displaystyle (S^{2}, d)\, } の理想点は 構文解析に失敗 (MathML、ただし動作しない場合はSVGかPNGで代替(最新ブラウザーや補助ツールに推奨): サーバー「https://en.wikipedia.org/api/rest_v1/」から無効な応答 ("Math extension cannot connect to Restbase."):): {\displaystyle {\rm M}^{1}\, } なので, 構文解析に失敗 (MathML、ただし動作しない場合はSVGかPNGで代替(最新ブラウザーや補助ツールに推奨): サーバー「https://en.wikipedia.org/api/rest_v1/」から無効な応答 ("Math extension cannot connect to Restbase."):): {\displaystyle f^{{\rm KS}}(S^{2}, d)=B\, } となる. しかし交渉領域が 構文解析に失敗 (MathML、ただし動作しない場合はSVGかPNGで代替(最新ブラウザーや補助ツールに推奨): サーバー「https://en.wikipedia.org/api/rest_v1/」から無効な応答 ("Math extension cannot connect to Restbase."):): {\displaystyle S^{3}\, } に広がると, 構文解析に失敗 (MathML、ただし動作しない場合はSVGかPNGで代替(最新ブラウザーや補助ツールに推奨): サーバー「https://en.wikipedia.org/api/rest_v1/」から無効な応答 ("Math extension cannot connect to Restbase."):): {\displaystyle f^{{\rm KS}}(S^{3}, d)=C\, } となり, 再びプレイヤー2の妥結点利得は減少する. これは公理1のためで, 構文解析に失敗 (MathML、ただし動作しない場合はSVGかPNGで代替(最新ブラウザーや補助ツールに推奨): サーバー「https://en.wikipedia.org/api/rest_v1/」から無効な応答 ("Math extension cannot connect to Restbase."):): {\displaystyle f^{{\rm KS}}(S^{0}, d)=C^{\prime}\, } 故に, 構文解析に失敗 (MathML、ただし動作しない場合はSVGかPNGで代替(最新ブラウザーや補助ツールに推奨): サーバー「https://en.wikipedia.org/api/rest_v1/」から無効な応答 ("Math extension cannot connect to Restbase."):): {\displaystyle f^{{\rm KS}}(S^{3}, d)=C\, } となるのである.
|
|
|||
| 交渉問題 | ナッシュ解 | 構文解析に失敗 (MathML、ただし動作しない場合はSVGかPNGで代替(最新ブラウザーや補助ツールに推奨): サーバー「https://en.wikipedia.org/api/rest_v1/」から無効な応答 ("Math extension cannot connect to Restbase."):): {\displaystyle {\rm KS}\, } 解 | 均等解 |
|
|
|||
| 構文解析に失敗 (MathML、ただし動作しない場合はSVGかPNGで代替(最新ブラウザーや補助ツールに推奨): サーバー「https://en.wikipedia.org/api/rest_v1/」から無効な応答 ("Math extension cannot connect to Restbase."):): {\displaystyle (S^{1}, d)\, } | 構文解析に失敗 (MathML、ただし動作しない場合はSVGかPNGで代替(最新ブラウザーや補助ツールに推奨): サーバー「https://en.wikipedia.org/api/rest_v1/」から無効な応答 ("Math extension cannot connect to Restbase."):): {\displaystyle {\rm A}\, } | 構文解析に失敗 (MathML、ただし動作しない場合はSVGかPNGで代替(最新ブラウザーや補助ツールに推奨): サーバー「https://en.wikipedia.org/api/rest_v1/」から無効な応答 ("Math extension cannot connect to Restbase."):): {\displaystyle {\rm A}\, } | 構文解析に失敗 (MathML、ただし動作しない場合はSVGかPNGで代替(最新ブラウザーや補助ツールに推奨): サーバー「https://en.wikipedia.org/api/rest_v1/」から無効な応答 ("Math extension cannot connect to Restbase."):): {\displaystyle {\rm A}\, } |
|
|
|||
| 構文解析に失敗 (MathML、ただし動作しない場合はSVGかPNGで代替(最新ブラウザーや補助ツールに推奨): サーバー「https://en.wikipedia.org/api/rest_v1/」から無効な応答 ("Math extension cannot connect to Restbase."):): {\displaystyle (S^{2}, d)\, } | 構文解析に失敗 (MathML、ただし動作しない場合はSVGかPNGで代替(最新ブラウザーや補助ツールに推奨): サーバー「https://en.wikipedia.org/api/rest_v1/」から無効な応答 ("Math extension cannot connect to Restbase."):): {\displaystyle {\rm C}\, } | 構文解析に失敗 (MathML、ただし動作しない場合はSVGかPNGで代替(最新ブラウザーや補助ツールに推奨): サーバー「https://en.wikipedia.org/api/rest_v1/」から無効な応答 ("Math extension cannot connect to Restbase."):): {\displaystyle {\rm B}\, } | |
|
|
|||
| 構文解析に失敗 (MathML、ただし動作しない場合はSVGかPNGで代替(最新ブラウザーや補助ツールに推奨): サーバー「https://en.wikipedia.org/api/rest_v1/」から無効な応答 ("Math extension cannot connect to Restbase."):): {\displaystyle (S^{3}, d)\, } | 構文解析に失敗 (MathML、ただし動作しない場合はSVGかPNGで代替(最新ブラウザーや補助ツールに推奨): サーバー「https://en.wikipedia.org/api/rest_v1/」から無効な応答 ("Math extension cannot connect to Restbase."):): {\displaystyle {\rm C}\, } | 構文解析に失敗 (MathML、ただし動作しない場合はSVGかPNGで代替(最新ブラウザーや補助ツールに推奨): サーバー「https://en.wikipedia.org/api/rest_v1/」から無効な応答 ("Math extension cannot connect to Restbase."):): {\displaystyle {\rm C}\, } | 構文解析に失敗 (MathML、ただし動作しない場合はSVGかPNGで代替(最新ブラウザーや補助ツールに推奨): サーバー「https://en.wikipedia.org/api/rest_v1/」から無効な応答 ("Math extension cannot connect to Restbase."):): {\displaystyle {\rm B}\, } |
|
|
|||
個人間効用比較が可能な交渉状況を考え, 公理1を要件としなければ, より強い単調性を満たす均等解 (egalitarian solution) が公理化される. 条件「基準点が等しい交渉問題 構文解析に失敗 (MathML、ただし動作しない場合はSVGかPNGで代替(最新ブラウザーや補助ツールに推奨): サーバー「https://en.wikipedia.org/api/rest_v1/」から無効な応答 ("Math extension cannot connect to Restbase."):): {\displaystyle (S, d)\, }
と 構文解析に失敗 (MathML、ただし動作しない場合はSVGかPNGで代替(最新ブラウザーや補助ツールに推奨): サーバー「https://en.wikipedia.org/api/rest_v1/」から無効な応答 ("Math extension cannot connect to Restbase."):): {\displaystyle (T, d)\, }
について, 構文解析に失敗 (MathML、ただし動作しない場合はSVGかPNGで代替(最新ブラウザーや補助ツールに推奨): サーバー「https://en.wikipedia.org/api/rest_v1/」から無効な応答 ("Math extension cannot connect to Restbase."):): {\displaystyle T\subseteq S\, }
ならば, 構文解析に失敗 (MathML、ただし動作しない場合はSVGかPNGで代替(最新ブラウザーや補助ツールに推奨): サーバー「https://en.wikipedia.org/api/rest_v1/」から無効な応答 ("Math extension cannot connect to Restbase."):): {\displaystyle f(T, d)\le f(S, d)\, }
」を単調性の公理と呼ぶと, 交渉問題の集合 構文解析に失敗 (MathML、ただし動作しない場合はSVGかPNGで代替(最新ブラウザーや補助ツールに推奨): サーバー「https://en.wikipedia.org/api/rest_v1/」から無効な応答 ("Math extension cannot connect to Restbase."):): {\displaystyle B_{E}\, }
上で, 公理2, 3, かつ, 単調性を満たす交渉解が一意に定まる. その交渉解は交渉領域内で各プレイヤーの基準点からの利得増加分を等しく最大化する点であり, 均等解と呼ばれる [2]. ただし, 考察する集合を構文解析に失敗 (MathML、ただし動作しない場合はSVGかPNGで代替(最新ブラウザーや補助ツールに推奨): サーバー「https://en.wikipedia.org/api/rest_v1/」から無効な応答 ("Math extension cannot connect to Restbase."):): {\displaystyle B_0\, }
とすると, 均等解は必ずしもパレート最適ではない. 以上3つの交渉解を図1の例によって整理すると, 表1のようになる.
2 交互オファーゲーム 交互オファーゲームは, 2人のプレイヤーが所与の価値の分配, 例えば, 分割可能な財1単位の分配について, 相手が了承するまで, 繰り返し交互に分配案を提示しあっていくゲームである.
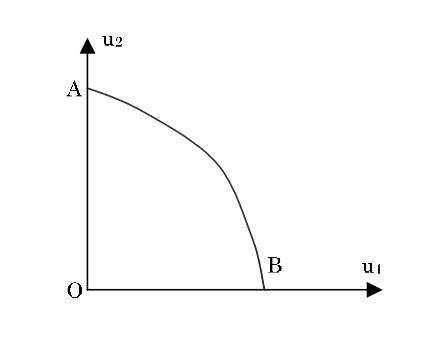
| 図2:財分配の実現可能集合 |
いま, 各プレイヤー 構文解析に失敗 (MathML、ただし動作しない場合はSVGかPNGで代替(最新ブラウザーや補助ツールに推奨): サーバー「https://en.wikipedia.org/api/rest_v1/」から無効な応答 ("Math extension cannot connect to Restbase."):): {\displaystyle i\, }
は, 構文解析に失敗 (MathML、ただし動作しない場合はSVGかPNGで代替(最新ブラウザーや補助ツールに推奨): サーバー「https://en.wikipedia.org/api/rest_v1/」から無効な応答 ("Math extension cannot connect to Restbase."):): {\displaystyle x\, }
単位の財から利得 構文解析に失敗 (MathML、ただし動作しない場合はSVGかPNGで代替(最新ブラウザーや補助ツールに推奨): サーバー「https://en.wikipedia.org/api/rest_v1/」から無効な応答 ("Math extension cannot connect to Restbase."):): {\displaystyle U_{i}(x)\, }
を得て, 利得関数 構文解析に失敗 (MathML、ただし動作しない場合はSVGかPNGで代替(最新ブラウザーや補助ツールに推奨): サーバー「https://en.wikipedia.org/api/rest_v1/」から無効な応答 ("Math extension cannot connect to Restbase."):): {\displaystyle U_{i}\, }
は連続狭義単調増加で凹, かつ, 構文解析に失敗 (MathML、ただし動作しない場合はSVGかPNGで代替(最新ブラウザーや補助ツールに推奨): サーバー「https://en.wikipedia.org/api/rest_v1/」から無効な応答 ("Math extension cannot connect to Restbase."):): {\displaystyle U_{i}(0)=0\, }
とする. すると, 2人に実現可能な利得の集合は, となり, 図2の曲線 構文解析に失敗 (MathML、ただし動作しない場合はSVGかPNGで代替(最新ブラウザーや補助ツールに推奨): サーバー「https://en.wikipedia.org/api/rest_v1/」から無効な応答 ("Math extension cannot connect to Restbase."):): {\displaystyle AB\, }
のようになる.
ゲームは次のように進行する. まず第1期に, プレイヤー1が, 分配案として, 集合 構文解析に失敗 (MathML、ただし動作しない場合はSVGかPNGで代替(最新ブラウザーや補助ツールに推奨): サーバー「https://en.wikipedia.org/api/rest_v1/」から無効な応答 ("Math extension cannot connect to Restbase."):): {\displaystyle P\, } 上の1点 構文解析に失敗 (MathML、ただし動作しない場合はSVGかPNGで代替(最新ブラウザーや補助ツールに推奨): サーバー「https://en.wikipedia.org/api/rest_v1/」から無効な応答 ("Math extension cannot connect to Restbase."):): {\displaystyle (u_{1}, u_{2})\, } をプレイヤー2に提示する. プレイヤー2が了承すれば, 分配案が実現してゲームは終了し, 却下した場合には, 第2期に入る. 以下, 次の期に入る毎にプレイヤーの役割が交代されて, 第1期と同様な手番でゲームが進行する.
2人は共通の割引率 構文解析に失敗 (MathML、ただし動作しない場合はSVGかPNGで代替(最新ブラウザーや補助ツールに推奨): サーバー「https://en.wikipedia.org/api/rest_v1/」から無効な応答 ("Math extension cannot connect to Restbase."):): {\displaystyle \delta\in(0, 1)\, } を持つとし, プレイヤー 構文解析に失敗 (MathML、ただし動作しない場合はSVGかPNGで代替(最新ブラウザーや補助ツールに推奨): サーバー「https://en.wikipedia.org/api/rest_v1/」から無効な応答 ("Math extension cannot connect to Restbase."):): {\displaystyle i\, } が, 第 構文解析に失敗 (MathML、ただし動作しない場合はSVGかPNGで代替(最新ブラウザーや補助ツールに推奨): サーバー「https://en.wikipedia.org/api/rest_v1/」から無効な応答 ("Math extension cannot connect to Restbase."):): {\displaystyle t\, } 期に利得 構文解析に失敗 (MathML、ただし動作しない場合はSVGかPNGで代替(最新ブラウザーや補助ツールに推奨): サーバー「https://en.wikipedia.org/api/rest_v1/」から無効な応答 ("Math extension cannot connect to Restbase."):): {\displaystyle u_{i}\, } を得た場合の現在利得は, であるとする. そして, これを交互オファーゲームの利得とする. 2人が永久に分配案を了承しない場合のゲームの利得は0とする.
ルビンシュタインは, この交互オファーゲームの部分ゲーム完全均衡利得は一意に定まり, 構文解析に失敗 (MathML、ただし動作しない場合はSVGかPNGで代替(最新ブラウザーや補助ツールに推奨): サーバー「https://en.wikipedia.org/api/rest_v1/」から無効な応答 ("Math extension cannot connect to Restbase."):): {\displaystyle \delta\rightarrow 1\, } のとき, 構文解析に失敗 (MathML、ただし動作しない場合はSVGかPNGで代替(最新ブラウザーや補助ツールに推奨): サーバー「https://en.wikipedia.org/api/rest_v1/」から無効な応答 ("Math extension cannot connect to Restbase."):): {\displaystyle u^*\, } は構文解析に失敗 (MathML、ただし動作しない場合はSVGかPNGで代替(最新ブラウザーや補助ツールに推奨): サーバー「https://en.wikipedia.org/api/rest_v1/」から無効な応答 ("Math extension cannot connect to Restbase."):): {\displaystyle P\, } の上で構文解析に失敗 (MathML、ただし動作しない場合はSVGかPNGで代替(最新ブラウザーや補助ツールに推奨): サーバー「https://en.wikipedia.org/api/rest_v1/」から無効な応答 ("Math extension cannot connect to Restbase."):): {\displaystyle u_1u_2\, } を最大にする点に収束することを証明した [1].
この結果から, 割引率が1に収束するとき, 均衡利得 構文解析に失敗 (MathML、ただし動作しない場合はSVGかPNGで代替(最新ブラウザーや補助ツールに推奨): サーバー「https://en.wikipedia.org/api/rest_v1/」から無効な応答 ("Math extension cannot connect to Restbase."):): {\displaystyle u^{*}\, } は, 集合 構文解析に失敗 (MathML、ただし動作しない場合はSVGかPNGで代替(最新ブラウザーや補助ツールに推奨): サーバー「https://en.wikipedia.org/api/rest_v1/」から無効な応答 ("Math extension cannot connect to Restbase."):): {\displaystyle P\, } をパレート最適集合に持つ協力実現可能集合と基準点が 構文解析に失敗 (MathML、ただし動作しない場合はSVGかPNGで代替(最新ブラウザーや補助ツールに推奨): サーバー「https://en.wikipedia.org/api/rest_v1/」から無効な応答 ("Math extension cannot connect to Restbase."):): {\displaystyle d=0\, } である交渉問題の, ナッシュ解の妥結点となることが分かる. つまり, 合意遅延のコストが十分小さい場合, 交互オファーゲームは, ナッシュ解の具体的交渉過程モデルの1つとなる. ナッシュプログラム参照)
ナッシュ解のみでなく, 構文解析に失敗 (MathML、ただし動作しない場合はSVGかPNGで代替(最新ブラウザーや補助ツールに推奨): サーバー「https://en.wikipedia.org/api/rest_v1/」から無効な応答 ("Math extension cannot connect to Restbase."):): {\displaystyle {\rm KS}\, } 解や均等解についても, その非協力ゲームモデルを与える研究が行われている. そして, 人交渉問題の交渉解や情報不完備な非協力交渉ゲームの研究も進んでいる [1], [2].
参考文献
[1] M. Osborne and A. Rubinstein, Bargaining and Markets, Academic Press, 1990.
[2] W. Thomson, "Cooperative Models of Bargaining," in Handbook of Game Theory with Economic Applications ed. by R. Aumann et al, 1992, vol. 2, 1238-1284.
[3] J. Nash, "The Bargaining Problem," Econometrica, 18 (1950), 155-162.