「《積分幾何学》」の版間の差分
細 ("《積分幾何学》" を保護しました。 [edit=sysop:move=sysop]) |
|||
| 1行目: | 1行目: | ||
'''【せきぶんきかがく (integral geometry)】''' | '''【せきぶんきかがく (integral geometry)】''' | ||
| − | + | “ビュッフォンの針”のように図形に関係した確率を幾何確率といい, これらの理論的な部分は, 積分幾何学(integral geometry)を基礎にしている. 積分幾何学は幾何確率に応用可能であるばかりでなく, もっと広く図形に関する様々な局面で基礎となり得ると考えられる. そこで積分幾何学の基礎概念と直線に関する主公式にかぎって述べることにする. 詳しくは[1], [2]を参照されたい. 積分幾何学の基礎概念とは合同変換によって不変な測度を求めることにあるが, 例を直観では分かりづらい直線の集合に採り, 以下で議論を展開する. | |
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| 21行目: | 11行目: | ||
\, </math> <math>(1)\, </math></center> | \, </math> <math>(1)\, </math></center> | ||
| − | と表される. そこで, この | + | |
| + | と表される. そこで, この<math>p</math>と<math>\theta \, </math>で, 直線の集合(たとえばある領域と交わる直線の集合など)の測度を | ||
| 31行目: | 22行目: | ||
| − | とおき, 以下, 合同変換で不変の条件を考えよう. 直線 (1)で表されるような, 直線の集合 <math>X\, </math> が, 合同変換 | + | とおき, 以下, 合同変換で不変の条件を考えよう. 直線 (1)で表されるような, 直線の集合 <math>X\, </math> が, |
| + | |||
| + | |||
| + | <center><table><tr><td align=center>[[画像:sk-0207-c-e-01-1.png]]</td></tr> | ||
| + | <td align=center>図1：直線に下ろした垂線<br><br>[[スタイル検討#積分幾何学 (0207-c-e-01-1)|スタイル検討]]</td></table></center> | ||
| + | |||
| + | <!-- | ||
| + | %\begin{figure}[h] %\begin{center} %%\vspace*{40mm} %%%%%%%%%%%%%%%%%%%%%%%%%% %\includegraphics{C-E-01f+2.eps} %\caption{直線に下ろした垂線} \label{fig:C-E-01+2} %\end{center} %\end{figure} | ||
| + | |||
| + | \begin{figure}[h] \begin{center} \begin{picture}(300,170)(-50,-20) %\put(-50,-20){\framebox(300,170)} \put(0,0){\vector(1,0){220}} \put(220,0){\makebox(0,0)[l]{\large $ x $ }} \put(0,0){\vector(0,1){130}} \put(0,130){\makebox(0,0)[b]{\large $ y $ }} \put(0,0){\line(1,2){50}} \put(-10,100){\line(2,-1){220}} \put(140,30){\makebox(0,0)[bl]{\large $ g $ }} \put(22,71){\line(2,-1){11}} \put(22,71){\line(1,2){5}} \put(20,50){\makebox(0,0)[tr]{\large $ p $ }} \put(0,0){\makebox(0,0)[tr]{O}} \put(5,0){\oval(15,20)[tr]} \put(15,15){\makebox(0,0)[l]{\large $ \theta $ }} \end{picture} \vspace{-10mm} \caption{直線に下ろした垂線} \label{fig:C-E-01+2} \end{center} \end{figure} | ||
| + | --> | ||
| + | |||
| + | |||
| + | 合同変換 | ||
| 52行目: | 56行目: | ||
| − | なる直線の集合 < | + | なる直線の集合 <math>X' \, </math> に変換されたものとする. すると, 上式より |
| 105行目: | 109行目: | ||
\int_{0}^{\pi}\sin\varphi \; \mbox{d}s \;\mbox{d}\varphi=2L\, </math> | \int_{0}^{\pi}\sin\varphi \; \mbox{d}s \;\mbox{d}\varphi=2L\, </math> | ||
</center> | </center> | ||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| 131行目: | 124行目: | ||
が導かれる. さて, これをもとに, 簡単な図形と交わる直線の集合の測度を求めよう. まず上記の曲線ABが直線分であれば, ほとんどいたるところ <math> n=1 \, </math> なので, 長さ <math> L \, </math> の線分と交わる直線の集合 <math> X \, </math> の測度は | が導かれる. さて, これをもとに, 簡単な図形と交わる直線の集合の測度を求めよう. まず上記の曲線ABが直線分であれば, ほとんどいたるところ <math> n=1 \, </math> なので, 長さ <math> L \, </math> の線分と交わる直線の集合 <math> X \, </math> の測度は | ||
| + | |||
| + | |||
| + | <center><table><tr><td align=center>[[画像:sk-0207-c-e-01-2.png]]</td></tr> | ||
| + | <td align=center>図２：曲線と直線<br><br>[[スタイル検討#積分幾何学 (0207-c-e-01-2)|スタイル検討]]</td></table></center> | ||
| + | |||
| + | <!-- | ||
| + | *{40mm} %\includegraphics{C-E-01+4.eps} %\caption{曲線と直線} \label{fig:C-E-01+4} %\end{center} %\end{figure} | ||
| + | |||
| + | \begin{figure}[h] \begin{center} \begin{picture}(300,170)(-50,-20) %\put(-50,-20){\framebox(300,170)} \put(0,0){\vector(1,0){220}} \put(220,0){\makebox(0,0)[l]{\large $ x $ }} \put(0,0){\vector(0,1){130}} \put(0,130){\makebox(0,0)[b]{\large $ y $ }} \put(0,0){\line(2,1){150}} \put(100,-10){\line(-1,2){70}} \put(90,10){\makebox(0,0)[bl]{\large $ g $ }} \put(71,22){\line(-1,2){5}} \put(71,22){\line(2,1){11}} \put(40,30){\makebox(0,0)[tr]{\large $ p $ }} \put(0,0){\makebox(0,0)[tr]{O}} \put(15,0){\oval(11,15)[tr]} \put(30,10){\makebox(0,0)[tl]{\large $ \theta $ }} \put(30,110){\oval(60,80)[br]} \put(56.2,77.6){\vector(2,3){40}} \put(56.6,85.5){\oval(9,8)[t]} \put(56,89.5){\makebox(0,0)[b]{\large $ \varphi $ }} \put(55.5,72){\makebox(0,0)[t]{\large P}} \put(30,70){\makebox(0,0)[r]{\large A}} \put(60,115){\makebox(0,0)[b]{\large B}} \end{picture} \vspace{-5mm} \caption{曲線と直線} \label{fig:C-E-01+4} | ||
| + | --> | ||
<center><math>m(X)=\int\mbox{d}G=2L\, </math> <math>(7)\, </math></center> | <center><math>m(X)=\int\mbox{d}G=2L\, </math> <math>(7)\, </math></center> | ||
| + | |||
| + | |||
| + | となる. 次に曲線が凸閉曲線の場合, ほとんどいたるところ <math> n=2 \, </math> が成立するので, 長さ <math> L \, </math> の凸閉曲線と交わる直線の集合 <math> X \, </math> の測度は | ||
| 144行目: | 150行目: | ||
\begin{figure}[h] \begin{center} \begin{picture}(300,140)(-50,-10) % \put(-50,-10){\framebox(300,140)} \put(-10,130){\line(2,-1){220}} \put(-30,130){\line(1,-1){130}} \put(40,50){\circle{80}} \put(90,60){\oval(200,100)} \put(150,90){\makebox(0,0)[bl]{\large $ C_0 $ }} \put(60,60){\makebox(0,0)[l]{\large $ C $ }} \end{picture} \vspace{-5mm} \caption{ $ C $ と交わる確率} \label{fig:C-E-01+5} \end{center} \end{figure} | \begin{figure}[h] \begin{center} \begin{picture}(300,140)(-50,-10) % \put(-50,-10){\framebox(300,140)} \put(-10,130){\line(2,-1){220}} \put(-30,130){\line(1,-1){130}} \put(40,50){\circle{80}} \put(90,60){\oval(200,100)} \put(150,90){\makebox(0,0)[bl]{\large $ C_0 $ }} \put(60,60){\makebox(0,0)[l]{\large $ C $ }} \end{picture} \vspace{-5mm} \caption{ $ C $ と交わる確率} \label{fig:C-E-01+5} \end{center} \end{figure} | ||
--> | --> | ||
| − | |||
| − | |||
| − | |||
2007年7月24日 (火) 11:07時点における版
【せきぶんきかがく (integral geometry)】
“ビュッフォンの針”のように図形に関係した確率を幾何確率といい, これらの理論的な部分は, 積分幾何学(integral geometry)を基礎にしている. 積分幾何学は幾何確率に応用可能であるばかりでなく, もっと広く図形に関する様々な局面で基礎となり得ると考えられる. そこで積分幾何学の基礎概念と直線に関する主公式にかぎって述べることにする. 詳しくは[1], [2]を参照されたい. 積分幾何学の基礎概念とは合同変換によって不変な測度を求めることにあるが, 例を直観では分かりづらい直線の集合に採り, 以下で議論を展開する.
図1のように, 直線 構文解析に失敗 (MathML、ただし動作しない場合はSVGかPNGで代替（最新ブラウザーや補助ツールに推奨）: サーバー「https://en.wikipedia.org/api/rest_v1/」から無効な応答 ("Math extension cannot connect to Restbase."):): {\displaystyle g\, }
があって, 原点からこれに下ろした垂線の長さを 構文解析に失敗 (MathML、ただし動作しない場合はSVGかPNGで代替（最新ブラウザーや補助ツールに推奨）: サーバー「https://en.wikipedia.org/api/rest_v1/」から無効な応答 ("Math extension cannot connect to Restbase."):): {\displaystyle p\, }
, 垂線と 軸との角度を 構文解析に失敗 (MathML、ただし動作しない場合はSVGかPNGで代替（最新ブラウザーや補助ツールに推奨）: サーバー「https://en.wikipedia.org/api/rest_v1/」から無効な応答 ("Math extension cannot connect to Restbase."):): {\displaystyle \theta\, }
とすると, この直線は
と表される. そこで, この構文解析に失敗 (MathML、ただし動作しない場合はSVGかPNGで代替（最新ブラウザーや補助ツールに推奨）: サーバー「https://en.wikipedia.org/api/rest_v1/」から無効な応答 ("Math extension cannot connect to Restbase."):): {\displaystyle p}
と構文解析に失敗 (MathML、ただし動作しない場合はSVGかPNGで代替（最新ブラウザーや補助ツールに推奨）: サーバー「https://en.wikipedia.org/api/rest_v1/」から無効な応答 ("Math extension cannot connect to Restbase."):): {\displaystyle \theta \, }
で, 直線の集合(たとえばある領域と交わる直線の集合など)の測度を
構文解析に失敗 (MathML、ただし動作しない場合はSVGかPNGで代替（最新ブラウザーや補助ツールに推奨）: サーバー「https://en.wikipedia.org/api/rest_v1/」から無効な応答 ("Math extension cannot connect to Restbase."):): {\displaystyle m(X)=\int_{X}f(p,\theta)\; \mbox{d}p \; \mbox{d}\theta \, } 構文解析に失敗 (MathML、ただし動作しない場合はSVGかPNGで代替（最新ブラウザーや補助ツールに推奨）: サーバー「https://en.wikipedia.org/api/rest_v1/」から無効な応答 ("Math extension cannot connect to Restbase."):): {\displaystyle (2)\, }
とおき, 以下, 合同変換で不変の条件を考えよう. 直線 (1)で表されるような, 直線の集合 構文解析に失敗 (MathML、ただし動作しない場合はSVGかPNGで代替（最新ブラウザーや補助ツールに推奨）: サーバー「https://en.wikipedia.org/api/rest_v1/」から無効な応答 ("Math extension cannot connect to Restbase."):): {\displaystyle X\, }
が,
 |
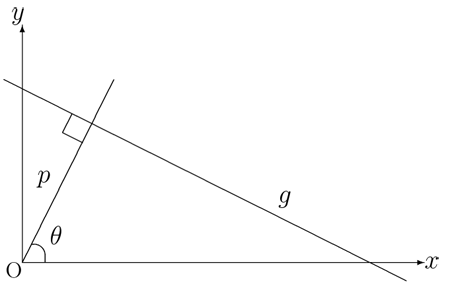| 図1：直線に下ろした垂線 スタイル検討 |
合同変換
構文解析に失敗 (MathML、ただし動作しない場合はSVGかPNGで代替（最新ブラウザーや補助ツールに推奨）: サーバー「https://en.wikipedia.org/api/rest_v1/」から無効な応答 ("Math extension cannot connect to Restbase."):): {\displaystyle \begin{array}{lll} x' &=& x\cos \alpha-\sin \alpha+a \\ y' &=& x\sin \alpha+y \cos \alpha+b \end{array}\, } 構文解析に失敗 (MathML、ただし動作しない場合はSVGかPNGで代替（最新ブラウザーや補助ツールに推奨）: サーバー「https://en.wikipedia.org/api/rest_v1/」から無効な応答 ("Math extension cannot connect to Restbase."):): {\displaystyle (3)\, }
によって,
構文解析に失敗 (MathML、ただし動作しない場合はSVGかPNGで代替（最新ブラウザーや補助ツールに推奨）: サーバー「https://en.wikipedia.org/api/rest_v1/」から無効な応答 ("Math extension cannot connect to Restbase."):): {\displaystyle x'\cos\theta'+y'\sin\theta'=p' \, }
なる直線の集合 構文解析に失敗 (MathML、ただし動作しない場合はSVGかPNGで代替（最新ブラウザーや補助ツールに推奨）: サーバー「https://en.wikipedia.org/api/rest_v1/」から無効な応答 ("Math extension cannot connect to Restbase."):): {\displaystyle X' \, }
に変換されたものとする. すると, 上式より
構文解析に失敗 (MathML、ただし動作しない場合はSVGかPNGで代替（最新ブラウザーや補助ツールに推奨）: サーバー「https://en.wikipedia.org/api/rest_v1/」から無効な応答 ("Math extension cannot connect to Restbase."):): {\displaystyle (4)\, }
が得られる. ここで, 一様な直線の分布を考えているので, 構文解析に失敗 (MathML、ただし動作しない場合はSVGかPNGで代替（最新ブラウザーや補助ツールに推奨）: サーバー「https://en.wikipedia.org/api/rest_v1/」から無効な応答 ("Math extension cannot connect to Restbase."):): {\displaystyle X\, }
と 構文解析に失敗 (MathML、ただし動作しない場合はSVGかPNGで代替（最新ブラウザーや補助ツールに推奨）: サーバー「https://en.wikipedia.org/api/rest_v1/」から無効な応答 ("Math extension cannot connect to Restbase."):): {\displaystyle X' \, }
の測度は等しく, すなわ
ち
でなければならない. ところが, 式 (4)から より 構文解析に失敗 (MathML、ただし動作しない場合はSVGかPNGで代替（最新ブラウザーや補助ツールに推奨）: サーバー「https://en.wikipedia.org/api/rest_v1/」から無効な応答 ("Math extension cannot connect to Restbase."):): {\displaystyle (p,\theta)\, }
への変数変換のヤコビアンは1なので, 上式の右辺を変形して
構文解析に失敗 (MathML、ただし動作しない場合はSVGかPNGで代替（最新ブラウザーや補助ツールに推奨）: サーバー「https://en.wikipedia.org/api/rest_v1/」から無効な応答 ("Math extension cannot connect to Restbase."):): {\displaystyle \int_{X}f(p,\theta)\; \mbox{d}p \; \mbox{d}\theta=\int_{X}f(p',\theta')\; \mbox{d}p \; \mbox{d}\theta }
と表すことができ, これは をどのようにとっても成立しなければならないので,
が得られる. そこで 構文解析に失敗 (MathML、ただし動作しない場合はSVGかPNGで代替（最新ブラウザーや補助ツールに推奨）: サーバー「https://en.wikipedia.org/api/rest_v1/」から無効な応答 ("Math extension cannot connect to Restbase."):): {\displaystyle c=1 \, }
とし, 構文解析に失敗 (MathML、ただし動作しない場合はSVGかPNGで代替（最新ブラウザーや補助ツールに推奨）: サーバー「https://en.wikipedia.org/api/rest_v1/」から無効な応答 ("Math extension cannot connect to Restbase."):): {\displaystyle \mbox{d}p \; \mbox{d} \theta \, }
を 構文解析に失敗 (MathML、ただし動作しない場合はSVGかPNGで代替（最新ブラウザーや補助ツールに推奨）: サーバー「https://en.wikipedia.org/api/rest_v1/」から無効な応答 ("Math extension cannot connect to Restbase."):): {\displaystyle \mbox{d}G\, }
とおいて, これを直線の集合 構文解析に失敗 (MathML、ただし動作しない場合はSVGかPNGで代替（最新ブラウザーや補助ツールに推奨）: サーバー「https://en.wikipedia.org/api/rest_v1/」から無効な応答 ("Math extension cannot connect to Restbase."):): {\displaystyle G \, }
の密度を表わすとすれば, 直線の集合の測度が式 (2)より
と導かれる. ここで, 図2のような滑らかな曲線ABがあり, その長さを 構文解析に失敗 (MathML、ただし動作しない場合はSVGかPNGで代替（最新ブラウザーや補助ツールに推奨）: サーバー「https://en.wikipedia.org/api/rest_v1/」から無効な応答 ("Math extension cannot connect to Restbase."):): {\displaystyle L \, }
とする. AB上の点Pでこの曲線と交わる直線を 構文解析に失敗 (MathML、ただし動作しない場合はSVGかPNGで代替（最新ブラウザーや補助ツールに推奨）: サーバー「https://en.wikipedia.org/api/rest_v1/」から無効な応答 ("Math extension cannot connect to Restbase."):): {\displaystyle g\, }
, 曲線ABのPにおける接線と 構文解析に失敗 (MathML、ただし動作しない場合はSVGかPNGで代替（最新ブラウザーや補助ツールに推奨）: サーバー「https://en.wikipedia.org/api/rest_v1/」から無効な応答 ("Math extension cannot connect to Restbase."):): {\displaystyle g\, }
とのなす角度を 構文解析に失敗 (MathML、ただし動作しない場合はSVGかPNGで代替（最新ブラウザーや補助ツールに推奨）: サーバー「https://en.wikipedia.org/api/rest_v1/」から無効な応答 ("Math extension cannot connect to Restbase."):): {\displaystyle \varphi \, }
, AからPまでの曲線上の長さを 構文解析に失敗 (MathML、ただし動作しない場合はSVGかPNGで代替（最新ブラウザーや補助ツールに推奨）: サーバー「https://en.wikipedia.org/api/rest_v1/」から無効な応答 ("Math extension cannot connect to Restbase."):): {\displaystyle s \, }
とおく. すると, 構文解析に失敗 (MathML、ただし動作しない場合はSVGかPNGで代替（最新ブラウザーや補助ツールに推奨）: サーバー「https://en.wikipedia.org/api/rest_v1/」から無効な応答 ("Math extension cannot connect to Restbase."):): {\displaystyle (p,\theta) \, }
から 構文解析に失敗 (MathML、ただし動作しない場合はSVGかPNGで代替（最新ブラウザーや補助ツールに推奨）: サーバー「https://en.wikipedia.org/api/rest_v1/」から無効な応答 ("Math extension cannot connect to Restbase."):): {\displaystyle (s,\varphi) \, }
への変数変換におけるヤコビアンは 構文解析に失敗 (MathML、ただし動作しない場合はSVGかPNGで代替（最新ブラウザーや補助ツールに推奨）: サーバー「https://en.wikipedia.org/api/rest_v1/」から無効な応答 ("Math extension cannot connect to Restbase."):): {\displaystyle |\sin\varphi| \, }
となり, 曲線ABと交わる直線のすべては, 構文解析に失敗 (MathML、ただし動作しない場合はSVGかPNGで代替（最新ブラウザーや補助ツールに推奨）: サーバー「https://en.wikipedia.org/api/rest_v1/」から無効な応答 ("Math extension cannot connect to Restbase."):): {\displaystyle 0\leq s\leq L, 0\leq\varphi \leq \pi \, }
の範囲を考えればよい. ゆえに
構文解析に失敗 (MathML、ただし動作しない場合はSVGかPNGで代替（最新ブラウザーや補助ツールに推奨）: サーバー「https://en.wikipedia.org/api/rest_v1/」から無効な応答 ("Math extension cannot connect to Restbase."):): {\displaystyle \int_{0}^{L}\! \int_{0}^{\pi}\sin\varphi \; \mbox{d}s \;\mbox{d}\varphi=2L\, }
となるので, この 構文解析に失敗 (MathML、ただし動作しない場合はSVGかPNGで代替（最新ブラウザーや補助ツールに推奨）: サーバー「https://en.wikipedia.org/api/rest_v1/」から無効な応答 ("Math extension cannot connect to Restbase."):): {\displaystyle 2L \, }
を曲線 構文解析に失敗 (MathML、ただし動作しない場合はSVGかPNGで代替（最新ブラウザーや補助ツールに推奨）: サーバー「https://en.wikipedia.org/api/rest_v1/」から無効な応答 ("Math extension cannot connect to Restbase."):): {\displaystyle AB \, }
と交わる直線の集合の測度 構文解析に失敗 (MathML、ただし動作しない場合はSVGかPNGで代替（最新ブラウザーや補助ツールに推奨）: サーバー「https://en.wikipedia.org/api/rest_v1/」から無効な応答 ("Math extension cannot connect to Restbase."):): {\displaystyle \textstyle \int\mbox{d}p \; \mbox{d}\theta \, }
としてよいかにみえる. ところが上記の積分は曲線 構文解析に失敗 (MathML、ただし動作しない場合はSVGかPNGで代替（最新ブラウザーや補助ツールに推奨）: サーバー「https://en.wikipedia.org/api/rest_v1/」から無効な応答 ("Math extension cannot connect to Restbase."):): {\displaystyle AB\, }
に沿って行なわれたので, この曲線と二つ以上の点で交わる直線については, それぞれの交点で計算されている. したがって, 上記の という値は求めたい測度よりも大きい数値であり, 構文解析に失敗 (MathML、ただし動作しない場合はSVGかPNGで代替（最新ブラウザーや補助ツールに推奨）: サーバー「https://en.wikipedia.org/api/rest_v1/」から無効な応答 ("Math extension cannot connect to Restbase."):): {\displaystyle n(p,\theta) \, }
でこの重複を表現すれば
が得られる. ところで, 曲線ABを滑らかなものとして議論してきたが, 滑らかな曲線を有限個つなげたものや, 不連続なものについても, ほとんどいたるところで接線は存在するので, 上式は成立する. そして対象となる曲線と交わらない直線については, 構文解析に失敗 (MathML、ただし動作しない場合はSVGかPNGで代替（最新ブラウザーや補助ツールに推奨）: サーバー「https://en.wikipedia.org/api/rest_v1/」から無効な応答 ("Math extension cannot connect to Restbase."):): {\displaystyle n(p,\theta)=0 \, }
と解釈し,
が導かれる. さて, これをもとに, 簡単な図形と交わる直線の集合の測度を求めよう. まず上記の曲線ABが直線分であれば, ほとんどいたるところ 構文解析に失敗 (MathML、ただし動作しない場合はSVGかPNGで代替（最新ブラウザーや補助ツールに推奨）: サーバー「https://en.wikipedia.org/api/rest_v1/」から無効な応答 ("Math extension cannot connect to Restbase."):): {\displaystyle n=1 \, }
なので, 長さ 構文解析に失敗 (MathML、ただし動作しない場合はSVGかPNGで代替（最新ブラウザーや補助ツールに推奨）: サーバー「https://en.wikipedia.org/api/rest_v1/」から無効な応答 ("Math extension cannot connect to Restbase."):): {\displaystyle L \, }
の線分と交わる直線の集合 構文解析に失敗 (MathML、ただし動作しない場合はSVGかPNGで代替（最新ブラウザーや補助ツールに推奨）: サーバー「https://en.wikipedia.org/api/rest_v1/」から無効な応答 ("Math extension cannot connect to Restbase."):): {\displaystyle X \, }
の測度は
 |
| 図２：曲線と直線 スタイル検討 |
となる. 次に曲線が凸閉曲線の場合, ほとんどいたるところ 構文解析に失敗 (MathML、ただし動作しない場合はSVGかPNGで代替（最新ブラウザーや補助ツールに推奨）: サーバー「https://en.wikipedia.org/api/rest_v1/」から無効な応答 ("Math extension cannot connect to Restbase."):): {\displaystyle n=2 \, }
が成立するので, 長さ 構文解析に失敗 (MathML、ただし動作しない場合はSVGかPNGで代替（最新ブラウザーや補助ツールに推奨）: サーバー「https://en.wikipedia.org/api/rest_v1/」から無効な応答 ("Math extension cannot connect to Restbase."):): {\displaystyle L \, }
の凸閉曲線と交わる直線の集合 構文解析に失敗 (MathML、ただし動作しない場合はSVGかPNGで代替（最新ブラウザーや補助ツールに推奨）: サーバー「https://en.wikipedia.org/api/rest_v1/」から無効な応答 ("Math extension cannot connect to Restbase."):): {\displaystyle X \, }
の測度は
 |
| 図３：構文解析に失敗 (MathML、ただし動作しない場合はSVGかPNGで代替（最新ブラウザーや補助ツールに推奨）: サーバー「https://en.wikipedia.org/api/rest_v1/」から無効な応答 ("Math extension cannot connect to Restbase."):): {\displaystyle C\, }
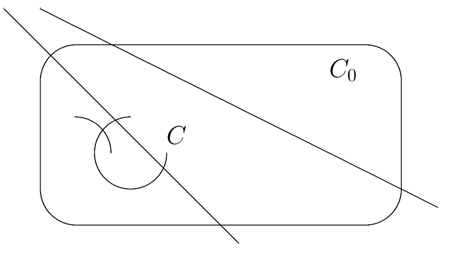と交わる確率 スタイル検討 |
となる. これを用いると, 以下のような確率を簡単に求めることができる. 図3のように凸閉曲線 構文解析に失敗 (MathML、ただし動作しない場合はSVGかPNGで代替（最新ブラウザーや補助ツールに推奨）: サーバー「https://en.wikipedia.org/api/rest_v1/」から無効な応答 ("Math extension cannot connect to Restbase."):): {\displaystyle C_0,C \, }
があって, 長さをそれぞれ, 構文解析に失敗 (MathML、ただし動作しない場合はSVGかPNGで代替（最新ブラウザーや補助ツールに推奨）: サーバー「https://en.wikipedia.org/api/rest_v1/」から無効な応答 ("Math extension cannot connect to Restbase."):): {\displaystyle L_0,L \, }
とする. 曲線 構文解析に失敗 (MathML、ただし動作しない場合はSVGかPNGで代替（最新ブラウザーや補助ツールに推奨）: サーバー「https://en.wikipedia.org/api/rest_v1/」から無効な応答 ("Math extension cannot connect to Restbase."):): {\displaystyle C_0,C \, }
に交わる直線の集合を 構文解析に失敗 (MathML、ただし動作しない場合はSVGかPNGで代替（最新ブラウザーや補助ツールに推奨）: サーバー「https://en.wikipedia.org/api/rest_v1/」から無効な応答 ("Math extension cannot connect to Restbase."):): {\displaystyle X_0,X \, }
とすれば, 構文解析に失敗 (MathML、ただし動作しない場合はSVGかPNGで代替（最新ブラウザーや補助ツールに推奨）: サーバー「https://en.wikipedia.org/api/rest_v1/」から無効な応答 ("Math extension cannot connect to Restbase."):): {\displaystyle C_0 \, }
に交わる直線が, 構文解析に失敗 (MathML、ただし動作しない場合はSVGかPNGで代替（最新ブラウザーや補助ツールに推奨）: サーバー「https://en.wikipedia.org/api/rest_v1/」から無効な応答 ("Math extension cannot connect to Restbase."):): {\displaystyle C\, }
にも交わる確率 は, 式(8)より
となる.
参考文献
[1] L.A.Santaló, Integral Geometry and Geometric Probability, Addison-Wesley, Reading, Massachusetts, 1976.
[2] 腰塚武志, 「積分幾何学について(1)～(4)」, 『オペレーションズ・リサーチ』, 21 (1976), 524-529, 591-596, 654-659, 711-717.